Moment of Inertia

by

Introduction

Moment of inertia functions in a manner similar to mass in linear motion but with rotating motion. Measurement of an object’s resistance to variations in its rotational motion along an axis is known as its moment of inertia.
It is more difficult to accelerate or deaccelerate an object’s rotation the greater its moment of inertia. Mass of an object and its distribution with respect to the rotational axis determine its moment of inertia.

Definition of Moment of Inertia

Moment of inertia, mathematically, is expressed as follow:-
                             I  = mi​ ri2​
Where:
I is the moment of inertia,
mi is the mass of the i particle,
ri is the perpendicular distance of the particle from the axis of rotation.
This equation represents the moment of inertia for a system of separate particles. For a nonstop body, the summation is replaced by an integral:                               I = r2dm
Where dm represents a small mass element of the object, and r is its distance from the axis of rotation.
It is a scalar quantity, the moment of inertia can also change depending on the object’s shape and the direction of the axis of rotation. Different moments of inertia about different axes may be experienced by the same object. When a rod is rotated about its center, for example, its moment of inertia will be different from when it is turned about one of its ends.

Factors Affecting Moment of Inertia

1.Mass of the Object: Object’s mass and moment of inertia are intimately co-related. With a higher moment of inertia, an object with greater mass will be more flexible to rotational shifts.
2. Distribution of Mass: Moment of inertia is primarily determined by the mass distribution with respect to the axis of rotation. Moment of inertia increases with the distance of the mass distribution from the axis. This explains why, when turned about its center, a thin rod has a smaller moment of inertia than when rotated about its end.
3. Shape of the Object: Moments of inertia varies among forms. A ring of the same mass and radius, for example, has a different moment of inertia than a solid disk. Common geometrical shapes, such as spheres, cylinders, and rods, have standard moments of inertia about particular axes for rigid bodies, and these moments are frequently utilised in computations.
4. Axis of Rotation: Moment of inertia can be strongly influenced by the orientation and location of the axis of rotation. This results in ideas that aid in calculating the moment of inertia about various axes, such as the parallel and perpendicular axis theorems.

Moment of Inertia for Common Shapes

1. Solid Sphere (about its center):                             I = 2 / 5 M R2
Where M is the mass and R is the radius of the sphere.
2. Solid Cylinder (about its central axis):                   I = 1 / 2 M R2
Where M is the mass and R is the radius of the cylinder.
3. Rod (about an axis through its center, perpendicular to its length):                                      I = 1 / 12 M L2
Where M is the mass and L is the length of the rod
4. Thin Ring (about an axis through its center, perpendicular to the plane of the ring):                                          I = M R2
Where M is the mass and R is the radius of the ring.
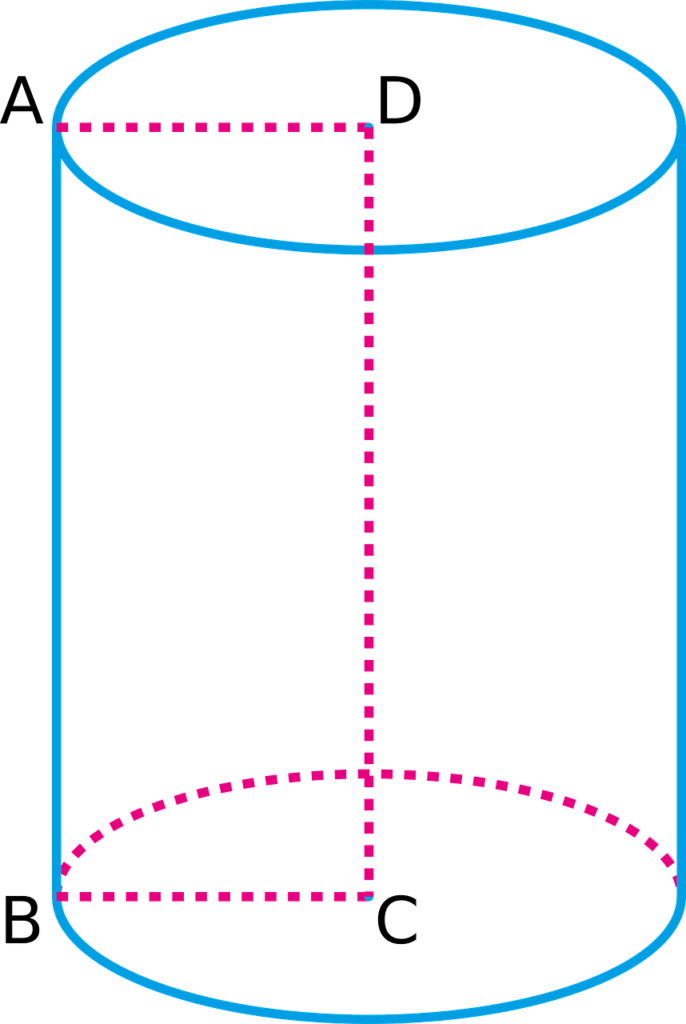
Moment of Inertia-Cylinder

Parallel Axis Theorem

Parallel axis theorem is used to find the moment of inertia of an object about an axis that is parallel to the axis through the center of mass. Theorem states:                                       I =  Icm + M d2
Where:
Icm ​ is the moment of inertia about the center of mass,
M is the mass of the object,
d is the distance between the center of mass axis and the new axis.
This theorem is particularly useful when calculating the moment of inertia for objects in systems where the axis of rotation does not pass through the center of mass.
Moment of Inertia- Ring

Perpendicular Axis Theorem

Perpendicular axis theorem is applicable to planar objects (flat objects). It states that the moment of inertia about an axis perpendicular to the plane of the object (denoted as Iz​) is equal to the sum of the moments of inertia about two perpendicular axes lying in the plane of the object (denoted as Ix and Iy):                                     Iz = Ix + Iy​
This theorem simplifies the calculation of moments of inertia for objects like disks or thin plates.

Importance in Physics and Engineering

Moment of inertia is vital, such as:
  • Rotational Kinetic Energy:  Rotational kinetic energy of a body is given by 1 / 2 I ω2, where ω is the angular velocity. The moment of inertia directly influences the energy associated with rotational motion.
  • Design of Mechanical Systems: Engineers consider moment of inertia when designing rotating components like flywheels, gears, and turbines to optimize their performance
  • Astrophysics: Distribution of mass and moment of inertia plays a significant role in the rotational dynamics of celestial bodies like stars and planets
Moment of Inertia-gear

Key sentences 

Mass rules linear motion, moment of inertia governs a better knowledge of circular motion. We can forecast an object’s behavior under rotational forces by examining its mass distribution and shape in relation to its axis of rotation. It is a vital idea in mechanics because of its applicability in both engineering and physics.
An object’s resistance to variations in its rotational motion about an axis is measured by its moment of inertia. It is dependent upon the mass of the item and its distribution with respect to the rotational axis. It is comparable to linear motion’s mass.
Moment of inertia for a rigid body is calculated using the integral formula:                               I = r2dm
where r is the distance of a mass element dm from the axis of rotation. For simpler systems, it can be summed as I = mi ri2​, where mi is the mass of each particle and ri is its distance from the axis.
Moment of inertia increases with mass distance from the axis of rotation. Moment of inertia of an object with mass concentrated close to the axis is less than that of an object with mass dispersed distant from the axis.
Parallel axis theorem states that the moment of inertia about any axis parallel to the one through the center of mass is given by:                                  I = Icm + M d2
where Icm is the moment of inertia through the center of mass, M is the object’s mass, and d is the distance between the two axes
Moment of inertia of a solid cylinder about its central axis is given by:                                   I = 1 / 2 M R2
where M is the mass of the cylinder and R is its radius.
Rotational kinetic energy of an object is given by:                                  K Erot = 1/ 2 I ω2
where I is the moment of inertia and ω is the angular velocity. A higher moment of inertia means the object will store more energy for the same angular velocity.
Yes, an object’s moment of inertia can change based on its rotational axis. A rod’s moment of inertia, for example, changes when it is spin around its center as opposed to one of its ends.

1 thought on “Moment of Inertia”

  1. Pingback: Measurement of Mass, methods important class 11

Leave a comment