Gas Laws: Boyle’s law, Charles’ law, gay Lussac’s law

by
Gas laws are describe how gases behave under different conditions of pressure, volume, and temperature. These laws are essential for understanding natural phenomena and have numerous applications in industries like medicine, engineering, and chemistry
Gas Laws-Gas
Gas

The Nature of Gases

Few basic properties of gases:
1.Compressibility: Gases can be compressed easily due to the large space between particles.
2.Expandability: They expand to fill any container, taking its shape
3. Low Density: Gases have much lower densities compared to solids and liquids.
4. Random Motion: Gas particles move randomly and collide with each other and the walls of the container.

Gas Laws

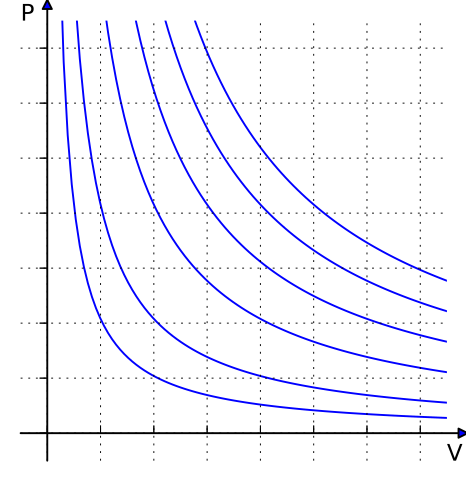
Boyle’s Law: Pressure and Volume Relationship

Boyle’s Law states:-

At constant temperature, the pressure of a gas is inversely proportional to its volume.
This means if we decrease the volume of a gas, its pressure increases, provided the temperature remains constant. Mathematically:
                                P ∝ 1 / V or P1V1 = P2V2​
Where:
  • P1​ and P2​ are the initial and final pressures.
  • V1​ and V2​ are the initial and final volumes.
Example- squeezing a balloon decreases its volume, increasing the internal pressure.
Pressure
Charles’s Law: Volume and Temperature Relationship

Charles’s Law states:

At constant pressure, the volume of a gas is directly proportional to its absolute temperature.

As the temperature of a gas increases, its volume also increases if the pressure is kept constant. Mathematically:

V∝T or V1 / T1 = V2 / T2 ​​

Where:
  • T is the temperature in Kelvin.
Example is the expansion of hot air in a hot-air balloon, which causes it to rise.

Gay-Lussac’s Law: Pressure and Temperature Relationship

Gay-Lussac’s Law states:

At constant volume, the pressure of a gas is directly proportional to its absolute temperature.
If the temperature increases, the pressure of the gas also increases.
Mathematically:
P ∝ T or P1 / T1 =  P2 / T2 ​​
This principle explains about pressure cookers effectiveness as higher temperatures inside the sealed cooker increase pressure and cooked the food faster.

Avogadro’s Law: Volume and Number of Molecules

Avogadro’s Law states:

At constant temperature and pressure, the volume of a gas is directly proportional to the number of moles of the gas.
Mathematically:
V ∝ n or V1 / n1= V2 / n2
Where:
  • n is the number of moles of the gas.
  • A gas follows Boyle’s law, Charle’s and Avogadro law strictly is called is an ideal gas.   
  • Such a gas is hypothetical. It is assumed that intermolecular forces are not present in between the molecules of an ideal gas.
  • Real gas follow these laws only under certain specific conditions when forces of interaction are practically negligible. In all other situations these deviate from ideal behaviour .
  • At STR P = 1 atm and T = 00 or 273 Kelvin
  • At SATR P = 1 bar and T = 298.15 Kelvin
  • At 1 atm pressure and 273 K 1 mole of a gas occupies 22.414 L
  • At 1 bar pressure and 273.15 K. 1 mole of gas occupies 22.711 L
This law explains about inflating a balloon with more air increases its size.
Gas laws-Molecules
Molecules

Ideal Gas Law

Combining all the Boyel’s , charle’s law and Avogadro law an equation can be derived whcih is known as the Ideal Gas equation.
V α 1 / P (constant T) (Boyle’s law)
V α T  (constant P) (Charles’ law)
V α n (constant P and T) (Avogadro’s law)
Combining eqns. (i), (ii) and (iii)
V α nT / P or V = R nT / P
So, PV / T = nR or PV = nRT
Where:
  • P = Pressure,
  • V = Volume,
  • n = Number of moles,
  • R = Universal gas constant (8.314 J mol−1 K−1)
  • T = Temperature in Kelvin.
Ideal gas law applies to hypothetical gases and provides a baseline for understanding of real gases.
Ideal gas law
Relationship between molar mass and density;-  Let m be the mass of gas in grams and M be the molar mass of gas,
We know n = m /M and PV = nRT So, PV = m . RT / M
P = m .RT / V.M  = dRT / M or M = dRT / P
dT / P = M / R , sine M and R are constant for a gas.
So, dT / P = constant
Therefore, d1 T1 / P1 = d2 T2 / P2
Dalton’s law of partial pressure :- The total pressure exerted by the mixture of non-reactive gases is equal to the sum of the partial pressures of individual gases.
PTotal = P1 + P2 + P3 +… (at constant T.V)
PDry gas = P Total – aqueous tension , (where aqueous tension is the pressure exerted by saturated water vapour.)

Partial pressure in terms of mole fraction :-

Pi = Xi = PTotal , where Pi and Xi are partial pressure and mole fraction of  ith gas respectively

Applications of Gas Laws

1.Weather Prediction: Gas laws help meteorologists to understand atmospheric pressure and temperature changes.
2. Industrial Processes: They are essential in designing pressure systems, refrigerators, and air conditioners.
3. Aerospace Science: Gas laws explain how air pressure and temperature affect flight and propulsion.

Limitations of Gas Laws

  • Real Gases: Real gases deviate from ideal behavior under high pressure or low temperature.
  • Non-Uniform Systems: Gas laws assume uniform distribution, which may not hold in dynamic conditions

Different values and units of gas constant (R)

Units of pressure

Units of volume

Values of gas constant (R)

Units of gas constant (R)

atm

L

0.0821

L atm K-1 mol-i

atm

cm3

82.1

Atm cm3 K-1 mol-i

dynes cm-2

cm3

8.314 x102

Ergs K-1 mol-i

dynes cm-2

cm3

1.987

Cal K-1 mol-i

Pa or N m-2

m3

8.31

K-1 mol-i

 

Note

Gas laws are explain about macroscopic properties of gases to molecular interactions. These laws are not only give knowledge of natural phenomena but also enables technological advancements that improve daily life. Whether it’s inflating a tire or studying the earth’s atmosphere, gas laws are everywhere.
Gas laws are scientific principles that describe the behavior of gases in terms of pressure, volume, temperature, and the number of gas molecules. They are important for understanding natural phenomena and have practical applications in industries like weather forecasting, aerospace, and chemical engineering.
Boyle’s Law states that at a constant temperature, the pressure of a gas is inversely proportional to its volume. Example, if you compress a balloon (reduce its volume), the pressure inside increases.
Charles’s Law states that at constant pressure, the volume of a gas is directly proportional to its temperature. A practical example is a hot-air balloon as the air inside is heated, it expands, causing the balloon to rise.
The Ideal Gas Law (PV = nRT) combines Boyle’s, Charles’s, and Avogadro’s laws. It provides a comprehensive equation to calculate a gas’s pressure, volume, temperature, or moles under ideal conditions.
According to Gay-Lussac’s Law, at constant volume, the pressure of a gas increases with its temperature. Example- in a sealed container  heating the gas increases its pressure, Thats why aerosol cans warn against exposure to high temperatures.
Avogadro’s Law states that at constant temperature and pressure, the volume of a gas is directly proportional to the number of moles of gas. This principle is useful to determine molecular compositions and balancing chemical reactions in gases.
Gas laws ideally describe hypothetical “ideal gases.” Real gases give a rough ideas as, these laws under normal conditions but deviate at high pressures and low temperatures due to intermolecular forces and finite molecular sizes. The deviations are accounted for using modifications like the Van der Waals equation

Leave a comment